
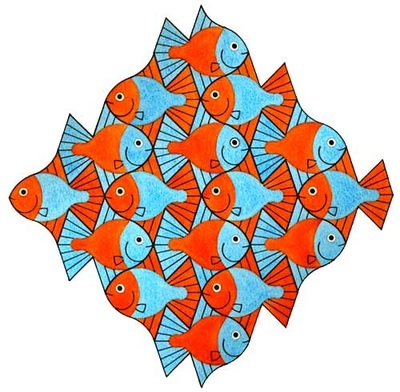
Some of the artwork is by children, some by adults, and some even by the past and present webmasters of. On this page, you'll find examples of art. Also, occasionally a tessellation fills a surface that isn't flat, like the outside of a soccer ball or a dodecahedron.

#Tessellation examples skin#
For example, that surface might be the outside of a ball, the outside of a lampshade, the inside of a decorated egg, the skin of a snake, a flat hexagon, a big wall, or a "circle limit". Occasionally, a tessellation fills a 2D surface that isn't an infinitely wide plane. We also prefer that the shapes' silhouettes resemble some other object: cat, camera, catfish, catapult, cabbage.anything that has a definite, recognizable shape. A tessellation looks a bit like a simple jigsaw puzzle, but all the pieces are just one or two or three shapes. The pattern fills, or clearly could fill, a surfaceĮntirely without gaps or overlaps". On this website, we define a tessellation as "a repetitive interlocking pattern of shapes.
#Tessellation examples how to#
How to Make an Asian Chop (stone stamp)Īccording to M.Escher, Penrose, and other "Recreational mathematicians. Look at American folk art that uses tessellations (such as quilts).Enter your class in one of several online tessellation contests.Use Web resources to extend the lesson:.There are examples from medieval European art as well (e.g., stained glass patterns). The earliest tessellations we can find come from Islamic art circa 3000 BC. Tessellations have been used all around the world for many years. If you can have students point out the three features of tessellations, it will help to make their understanding more concrete and it will also review the definition. Teach students about the history of tessellations and show examples.Encourage students to experiment to see if they can discover other ways to make shapes tessellate.They will notice that only some, not all, can make a pattern that would fit all three of the criteria. Younger students can discover for themselves what shapes tessellate using pattern blocks and lots of space.Have your students teach another class how to tessellate.(For example, ask them to tell you who is adjacent to them or ask them to label the top right vertex of a shape you provide.) To assess an understanding of the vocabulary, create a quiz, or ask them to perform another project that requires an understanding of the terms.Note how the students follow multi-step directions as well as how they cut and trace (manual dexterity).(These were chosen because each tessellates.) Using the Student Directions worksheet, demonstrate how to transform a shape into something that will also tessellate. Provide students with the Shapes worksheet within the Tessellations packet, which has a copy of a square, a rectangle,a rhombus, and a hexagon on it. Define plane (use a concrete example in the room) and show students how the pattern could continue on that plane if it were to go on beyond the confines of the building (e.g., it could continue as a pattern on the ceiling without any gaps or overlaps even if the ceiling were to continue forever, far beyond the walls of your school).Ģ. Third, tessellations can continue on a plane forever.If students have pointed to a pattern in the room that has a gap or an overlap in it, point out that it does not fit the definition of a tessellation. Second, tessellations do not have gaps or overlaps.Tell students that while those are repeated patterns, only some are tessellations because tessellations are a very specific kind of pattern. Generate a list of the words one could use to describe these patterns. Ask students to find examples of repeated patterns in the room. Discuss the three basic attributes of tessellations: Ask students to tell you what they know about the word tessellation. Introduce key vocabulary words: tessellation, polygon, angle, plane, vertex and adjacent. Scissors, tape, 11" x 14" paper, crayons, black fine-tip penġ.create a concrete model of a tessellation.be able to understand and define the following terms: tessellation, polygon, angle, plane, vertex, and adjacent.Escher, his art, or the contributions he made to mathematics. have the opportunity to go beyond the immediate lesson and apply artistic creativity, or learn more about M.This geometry lesson is integrated with history and art to engage even the most math resistant of your students and to enlighten everyone about M. The connections between art and math are strong and frequent, yet few students are aware of them.


 0 kommentar(er)
0 kommentar(er)
